METHODOLOGY TO DETERMINE THE INSTALLED CAPACITY OF AN ACADEMIC PROGRAM¹

PABLO CÉSAR MANYOMA VELÁSQUEZ*¹, JUAN PABLO OREJUELA CABRERA², CRISTIAM ANDRÉS GIL GONZÁLEZ³

¹Full time teacher, Escuela de Ingeniería Industrial y Estadística, Universidad del Valle, Colombia. pablo.manyoma@correounivalle.edu.co

²Full time teacher, Escuela de Ingeniería Industrial y Estadística, Universidad del Valle, Colombia. juan.orejuela@correounivalle.edu.co

³Young researcher, Escuela de Ingeniería Industrial y Estadística, Universidad del Valle, Colombia. Cristiam.gil@gmail.com

* Autor para correspondencia. Dirigir correspondencia a: Universidad del Valle, Calle 13 No. 100-00 Ed. 357 Of. 2007-2, Cali, Colombia.

Fecha de recepción: 18-05-2010 Fecha de corrección: 28-01-2011 Fecha de aceptación: 03-10-2011

ABSTRACT

Measuring capacity is a major problem, especially in service organizations because of its complexity and the lack of research on this topic at these kinds of organizations. Therefore, the purpose of this article is to discuss a methodology for measuring the capacity of an university by focusing on an academic unit. To this end, this research study determines the available capacity of an academic program and the use of resources by a typical student, and then the relation between these two aspects. The proposal is validated for an undergraduate program, generating a fairly good estimate of the number of students who can be served at any given time.

KEYWORDS

Capacity, capacity planning, university, service measurement, methodology.

JEL classification: I21, M11

RESUMEN

Metodologia para determinar la capacidad instalada en un programa academico

La medición de la capacidad es un problema de gran importancia, especialmente en las organizaciones de servicios dada su complejidad y la poca investigación realizada al respecto. En este sentido, el presente artículo tiene como objetivo desarrollar una metodología para la medición de la capacidad de una universidad desde la particularidad de una unidad académica. Para ello se determina cuál es el consumo de recursos de un estudiante típico, cuál es la capacidad instalada de los recursos y cuál es la relación existente entre estos dos elementos. La propuesta es validada en un programa de pregrado, generando una aproximación muy buena al número de estudiantes que pueden ser atendidos en un momento dado.

PALABRAS CLAVE

Capacidad, planificación de la capacidad, universidad, medición del servicio, metodología.

RESUMO

Metodologia para determinar a capacidade instalada em um programa acadêmico

A medição da capacidade é um problema de grande importância, especialmente nas organizações de serviços dada sua complexidade e a pouca investigação realizada a esse respeito. Nesse sentido, este trabalho tem como objetivo desenvolver uma metodologia para a medição da capacidade de uma universidade a partir da particularidade de uma unidade acadêmica. Para isso se determina qual é o consumo de recursos de um estudante típico, qual é a capacidade instalada dos recursos e qual a relação entre esses dois elementos. A proposta é validada em um programa de graduação, gerando uma ótima aproximação ao número de alunos que podem ser atendidos em um determinado momento.

PALAVRAS CHAVE

Capacidade, planificação da capacidade, universidade, medição do serviço, metodologia.

INTRODUCTION

Higher education systems in Latin America have undergone a true transformation due to globalization, new information and communications technologies, new demands for access on the part of the population, and the growing presence of knowledge societies. These factors have generated a mass production of college education. In the early 1990’s, the coverage rate was approximately 15% of the population (in the ages from 20 to 24). At the present time it is nearly 30% (UNESCO, 2005).

In these countries, 65% of higher education is provided by the private sector and 35% by the public sector (Dridriksson, 2008). Private university education has become, in many cases, the only option for most of the population.

In Colombia, there are 282 higher education institutions with the following general characteristics (Ministerio de Educación Nacional –MEN).²

• 81 are public and 201 are private universities.
• 79 are universities; the others are institutions of minor importance.
• 39% are located in Bogota, 14% in Antioquia, 10% in Valle del Cauca, and 37% are distributed in the remaining 24 states.
• In the last 8 years, enrollment has increased by 52%, the number of admission applications by 26%, and the number of first semester students by 63%.

For the Colombian government measuring capacity at each of the institutions in the public university system has clearly become a goal because of three essential aspects of the service, namely, coverage, quality, and efficiency. Since 2005, the National Ministry of Education (NME or MEN, because of its Spanish acronym) has developed projects together with public universities in order to achieve this goal under the following premise: there is a need to have an estimate of the installed capacity of each public university in order to be able to negotiate with them the setting of goals for the future. This can also be an instrument for the Ministry to determine the limits of growth and expansion of the education sector without affecting optimal levels of efficiency (MEN, 2005).

From the perspective of public universities, the efforts on the part of the national government have primarily translated into an opportunity to obtain a financial profit due to the enactment of law 715 from 2001 (Overall Participation System). This law establishes an equitable allocation of educational resources based on criteria such as, e.g.: number of students, dispersion of the population, share of admitted students, and teaching and administrative staff costs (MEN, 2004). As a public higher education institution, Universidad del Valle is no outsider to these new policies.

1. UNIVERSIDAD DEL VALLE

1.1. General overview

Valle del Cauca represents one of the most important regions in terms of higher education since it has 8% of all the enrolled students in Colombia. In this region, the Universidad del Valle stands out because of the great contribution it has made to higher education throughout its 65 years of history. Until 2008, it accounted for approximately 66% of all regional admission applications, 26% of enrolled students and 44% of graduating students in the region (authors’ calculations using MEN data).

Universidad del Valle is composed of 7 faculties and 2 institutes, which offer about 70 different academic programs. It has one main campus and 9 regional facilities, reaching in 2008, a student population of 30.320. Comparing this value with the same figure at the start of 2000 (17.150), there was a 77% increase in the number of enrolled students. At the main campus in Cali, Colombia, the number of students in 2008 was 21.700, representing 78% of the university’s students (Universidad del Valle, 2009).

Despite its efforts to become an institution that offers quality education which is within the population’s reach, its rate of absorption, understood as the ratio of demand to the number of admitted students, is only around 36% (Instituto Latinoamericano de Liderazgo, 2010).

This ratio together with the current physical resources urges the need to determine the actual capacity (measured in the number of students) that the institution can provide adequately.

1.2. The problem

The planning, programming, and control of capacity in relation to the strategic planning of an organization are essential for formulating achievable and feasible goals on a short, medium, and long-term basis (Kalenatic, 2001).

Not having a reliable estimate of capacity leads the Universidad del Valle to make decisions on the allocation and use of resources and serve the demand based on an underestimated or overestimated capacity.

An educational institution is to ensure availability of all the resources that its students could possibly use. The main resources that a university provides include: classrooms, teaching hours, laboratory facilities, libraries, cafeterias, sports centers, medical services, ICT equipment, etc.

This case study only takes into account the classroom resource and addresses the following questions:

• What is the use capacity of a student like?
• What is the available capacity at the university for this resource?

The problem of determining the capacity is complex since there is no specific methodology for these cases.

2. MEASUREMENT OF CAPACITY

2.1. Definition

Capacity planning is aimed at determining the most appropriate amount of resources that should be offered to achieve an expected service level, so as to maximize the cost-benefit ratio.

Capacity planning also implies determining the use by each product or service unit of each resource, the capacity that every resource provides the current load of the system, demand forecasts for products or services on the planning horizon, and service level expectations (Kalenatic, 2001).

2.2. capacity in education

One of the roles of any local or national government is to provide certain goods and services that ensure wellbeing. Education is one of the most important services for the development of any country; this can be seen in the national budgets earmarked to this sector. Schools, colleges, universities, and learning centers are physical infrastructures used for producing this kind of service and, together with teachers, who are the workforce of this process, are the most important production factors in the education sector (Antunes and Peeters, 2000).

From the perspective of an educational institution, capacity can be determined as the maximum limit that an operating unit can keep. An operating unit is defined as faculties, schools, classroom spaces, laboratory facilities available, administrative staff, professorial staff and technological tools, computers, and literature, among others (Johnson, 2001).

With regard to educational facilities, the literature contains a large number of proposals on capacity investment: opening, closing, or expansion. Some of the authors engaged in this kind of work include: Greenleaf and Harrison (1987), Henig and Gerschak (1986), Pizzolato (1994), Tewari and Jena (1987), and Viegas (1987). But no one is seeking to approach capacity in terms of outputs; they have done this from inputs as a proportion of previously built infrastructure.

Institutions have a limited number of teaching spaces and resources that must be used efficiently. This efficiency is generally referred to as "utilization" which is basically the fraction of the hours-chair or hoursresource that are currently in use (Burgess, 1996).

In universities, the focus is usually to fit a course into a classroom over a period of time, but in many cases this is possible only if the course is split into several class sessions. Another common problem is when hundreds of students enroll in a subject; they are often divided into types and sizes according to the pedagogical requirements of the particular subject (Beyrouthy, Burke, Landa-Silva, McCollum, McMullan and Parkes, 2006).

Studies of capacity determination in educational institutions usually explore the capability of departments or cost centers without delving into the processes. The problem is that departments are broken down into more specific specialties and disciplines, which are often rejected by the hierarchical nature of aggregate planning (Burgess, 1996).

2.3. requirements for measuring

Capacity is generally defined as a set of resources used for creating customer value. According to Cox, Blackstone and Spencer (1995), it is defined as the amount of work that a particular resource can perform (employee, machine, work center, plant or organization) in a specific period of time.

Defining a measurement unit is the starting point to capacity planning (Orejuela, Ocampo and Mican, 2010). For Yu-Lee (2002) any item that can be measured in terms of capacity can be classified into one of the basic components listed below:

• Space. It represents the availability of physical locations that an organization has assigned for operation. For example, manufacturing facilities, offices, distribution centers, and the space available for transporting goods, among others. It is the space represented by any element that can be measured in terms of area or volume (square or cubic meters).
• Labor. It represents the amount of work done by an organization to carry out its operations. Work is usually measured as the amount of work done by one or more individuals in a standard period of time. Time is the main unit of measure used for managing work.
• Equipment. This refers to the machinery used for performing various operations at an organization, whether manufacturing or service providers. Each piece of equipment has its own capacity which is determined by the speed multiplied by the number of operating hours. However, the capacity of operation depends on the configuration of manufacturing systems as this determines the bottleneck in operations.
• Information technology. It represents the ability of an organization's computing resources to handle various types of data and information. From the perspective of networks, capacity is the amount of data that can be transmitted per unit of time. From the perspective of storage capacity, it represents the amount of data that can be saved. From the perspective of processing, it is the number of transactions or tasks that can be carried out in a certain period of time.
• Materials. This type of capacity is the amount of inventory available at an organization in order to anticipate demand. Theoretically, the amount of materials of an organization and the number of units of finished product determine its capacity.

According to Dominguez, Alvarez, Dominguez and Santiago (1995) capacity can be defined from two different perspectives: outputs such as the amount of product or service to be obtained for a unit of production in a period of time, adapting to the demand fluctuations that must be satisfied in the future. From the perspective of inputs, for example, it is the number of man-hours available.

From the perspective of outputs, it implies the presence of a single product or set of products with similar characteristics (as can be seen from resource consumption and demand behavior), so they can be added as a family of products and the obtained results are representative of the system. Determining consumption of capacity becomes a much more complex matter since there are products or services with varying time consumptions and with different demand behaviors, which makes it difficult to aggregate them into families. Besides it is not possible to define capacity in terms of each single product.

Since it is not possible to establish the capacity to provide products or services, because they compete for resources with each other, a combination of products must be defined according to a definition of strategic objectives. However, given that demand for the products doesn’t behave similarly, the mix changes at each point in time, thus making it difficult to define capacity in the medium term.

From the perspective of inputs, it is defined using the most representative or restricted inputs. Consumption capacity should be determined in terms of the input unit measurement. Thus, total capacity is subordinated to this input. Such an approach, by not including the outputs in its definition, facilitates the process, but does not resolve the question of how many specific products or services can be met in a time period. It also presents some difficulties when defining the most restricted resourced, because this definition implicitly depends on the behavior of demand for the products (mobile bottleneck).

On the other hand, by not providing the amount for each of the products or services, it is difficult to define the demand to meet because this, in principle, is expressed in units of specific products or services. Therefore, it is necessary to convert demand into units of resource consumption, and then compare them. In the case where the required load exceeds capacity, the definition of a specific number of products is not clear.

In summary, both approaches have their advantages and disadvantages; each has a more appropriate configuration depending on the characteristics of the system under study. For services systems, the definition of capacity is very common from the point of view of inputs, which is perfectly valid for services that consist of offering a single type of resource and various outputs; there is only a difference in time consumed in that resource.

In services environments with multiple resources and different outputs, the definition of capacity from the standpoint of resources and outputs cannot be applied directly due to the heterogeneity of products and demands. Defining planning and measuring strategies that allow good approximations is of utmost importance in the case of multiple services and multiple resources. These strategies must include the ability to measure capacity from outputs and inputs (Gil and Rivadeneira, 2008).

3. METHODOLOGY FOR MEASURING CAPACITY OF AN ACADEMIC PROGRAM AND A CASE STUDY

This research initiative is focused on the behavior of capacity at Universidad del Valle (overall level) from the perspective of an academic program.

The Industrial Engineering program began in September 1976, and since then it has had annual student admissions (every August). From January 2003, it began semiannual admissions, given the aforementioned situation about demand. Graph 1 below shows the evolution of the student population, increasing from 288 students in 2002 to 501 students in 2008.

This 74% increase in the number of students served did not necessarily mean an increase in infrastructure investment. On the contrary, it has raised problems with regard to the number of students per course, physical space, and teacher allocations. In order to continue to maintain the level of quality that the university has offered until now, it is necessary to clearly identify the actual capacity of the academic program (see Graph 2).

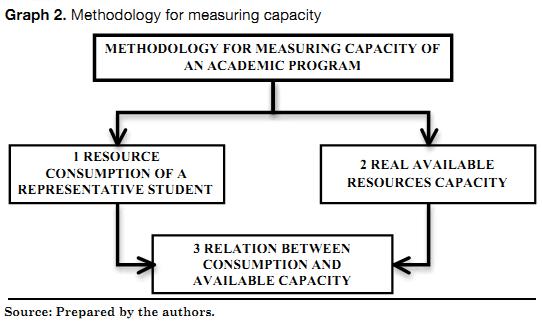
Three fundamental aspects were identified for the development of the methodology (see Graph 2):

• Identifying and defining a representative student's resource consumption;
• Determining the real available capacity of resources; and
• Identifying the relation between consumption and capacity.

3.1. Identification and definition of a representative student’s resource consumption

A representative student is an individual standard, which epitomizes the average behavior of the student population. This behavior is reflected on the enrollment process for subjects from different semesters.

To find the capacity consumption of a representative student (F_j), two elements are needed, namely, a) the time consumption of classroom resource for a representative student in a semester m (Q_m), and b) the quantity of representative students enrolled in semester m (X_m), as shown in Equation 1 below.

a) The time consumption of classroom resource for a representative student in semester m (Q_m) is determined by the consumption generated by the set of courses that belong to semester i (m), and the number of students who may enroll.

Table 1 showed the results of variable Q_m for the academic program in review. The third semester is taken as an example to illustrate the origin of the calculation.

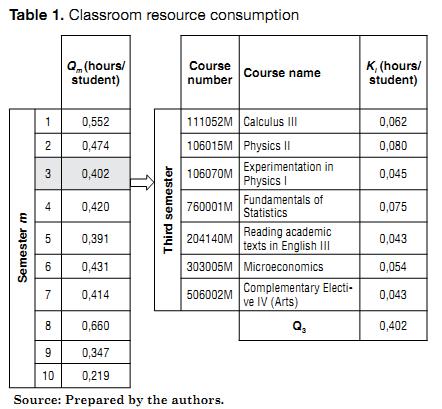
The weekly time of classroom resource consumed by a representative student, who is enrolled in subject i, is named Unitary load (Ki), this estimation assumes that group sizes are equal for each subject.

Where:

• Time needed T_i represents the weekly hours required by a teacher in a classroom to teach all course sessions for subject i.
• Group size G_i represents the number of students allowed in each group scheduled for subject i.
• Effective enrollment rate H_i is the rate of students enrolled in subject i with respect to all those who were eligible for enrollment.

Table 2 shows the necessary information to get the Unit load value for each subject who is in the third semester. From this point on, Physics II will be the subject used for the next calculations.

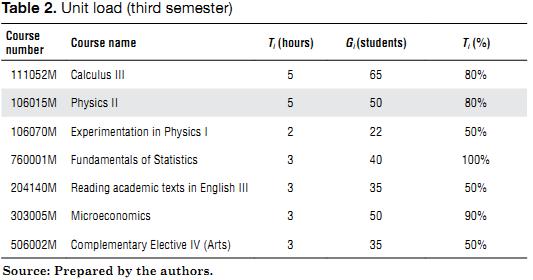
The effective enrollment rate is defined by Equation 4 below.

The enrolled students (M_i) are the number of students registered (in the information system) to attend subject i, while Apt Students (L_i) are the number of students who meet the requirements to enroll in a subject in the next period.

Table 3 shows the data which makes up the formula to calculate the Effective enrollment rate (H_i).

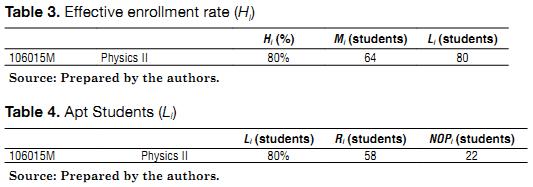
To calculate Apt Students L_i, Equation 5 is used. An example of this is presented in Table 4.

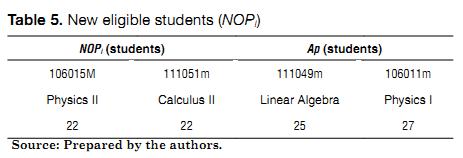
Where failed students (R_i), represents the number of students registered at the end of the period who do not pass subject i; and new eligible students (NOP_i) represents the number of students who can enroll in subject i for the first time, meaning they have completed and passed all prerequisite courses in the period (Equation 6).

Ap is the number of students who have passed course p that is a prerequisite for subject i,P(i). For example, the prerequisite courses for Physics II are Calculus II, Linear algebra, and Physics I, each of which had 22, 25 and 27 passing students, respectively (see Table 5), The minimum value for this set of subjects (22 students) will be the input for Physics II.

b) The number of representative students registered in semester m (X_m) is the following:

E_i is the expected number of students given the initial parameters for subject i who will not enroll in the following period:

Where , is the estimate of E_i, in the immediately preceding period.

Likewise, for the calculation of students per semester it is necessary to find the Expected Number of Registered Students (S_i) that represent the total number of students who, given the initial parameters for subject i, are consuming teacher and classroom resources. It corresponds to a steady state calculated with the convergence of function S_i:

The Desertion Rate (TD_m) is a percentage that reflects the historical behavior of those students who began their studies, but for several reasons decided to drop out (see Table 6).

The information shown in Table 7 is the result of a simulation of 20 admission processes, in other words, 35 years of operation. The initial values for S_i and E_i are 0 because there were no students enrolled at the beginning.

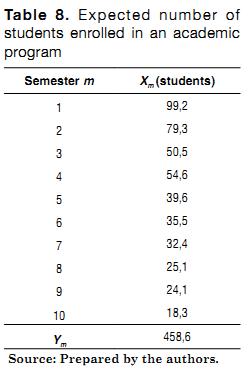
The number of representative students registered in semester m (X_m) is useful for determining the expected number of students enrolled in academic program j (Y_j) . For the Industrial Engineering program, the results (459 students) are shown in Table 8 and the relevant equation is as follows:

3.2. Determining the actual available capacity of resources

Up until now, the consumption of resources by a representative student in an academic program has been calculated. It is essential to identify the actual physical capacity of the university, measured by the number of classroom hours.

Consolidated classroom resource is given by:

Where:

• NDD are the week days available to schedule classes. A total of 5,5 days is estimated, from Mondays through Fridays on a full time basis, and Saturdays on a part-time basis.
• Threshold Time (MBR). Working hours at Universidad del Valle is the time during which the classrooms are used only for academic activities. This totals 13 hours, from 7 am to 9 pm minus an hour for lunch.
• Classroom utilization (UT). It is the rate of hours used for teaching a course divided by total available hours. It is compounded by all the hours scheduled into the classrooms plus the hours that could be scheduled. This parameter was estimated at around 82,3%.
• C_zj is the number of type z classrooms available for academic program j. It is composed of classrooms available for use by any academic program (O_z) and classrooms that are only be available for academic programs belonging to the same school (U_z). TC_j is the percentage which pertains to the industrial engineering program. The values are shown below (see Table 9).

The size of the chairs that can be placed in each classroom defines its type z. This methodology took into account three different types: spaces with a capacity of around 20, 35 and 55 chairs, respectively.

After finding the above parameters, Consolidated classroom resource (CSR_j) was estimated at 196,2 hours.

3.3. relation between consumption and capacity

Actual capacity determined in representative students (SQ_j) epitomizes the total number of users served by academic program j with all the classroom resource assigned to itself. It is defined by Equation 12 below.

Since the capacity consumption of a representative student (F_j) value is 0,455 hours/student and the Consolidated classroom resource (CSR_j) value is 196,2 hours, then SQ_j is equal to 431 students.

In steady state, the variable Students enrolled in the Academic Program j (Y_j) is the same as the variable Real Capacity determined in representative Students (SQ_j) in the actual state. Because of this, a comparison of these two variables could lead to the following conclusions:

• If Y_j>SQ_j, then academic program j does not have or will not have the necessary infrastructure to serve its student population. It requires short-term investments and measures or strategies to sustain service levels and prevent deterioration of the quality of teaching.
• If Y_j≈SQ_j, then the use of available infrastructure is sufficient to satisfy the requirements of capacity consumption of students enrolled in the academic program j. There is a need to analyze the tendency to make decisions regarding the future evolution of available capacity.
• If Y_j < SQ_j, then the academic program j is underusing the number of hours of classroom resource available for itself, possibly in favor of academic quality or out of ignorance. The advice given is to check the reasons and adopt corrective measures that allow for a better balance of the given available capacity to other academic programs.

A comparison between the numbers produced by the consumption behavior of the system capacity (Y_j), namely, 459 students, and the availability of resources (SQ_j), namely, 431 students, reveals that at the present time, the industrial engineering program is at its limits of occupancy. It is not going to have the necessary infrastructure to serve its student population if the current admission rates or current facilities (classrooms) continue to increase.

4. CONCLUSIONS

The methodology introduces the concept of student "average" as a way to consolidate the behavior of capacity consumption of student population. This behavior is reflected on the variability of the student enrollment process.

The scheduling problem is a field of knowledge that affects the capacity installed in any system. This topic is not addressed in this research study which does take into account its influence through the use of classroom setting (UT). This is a useful approach that helps determine the actual capacity of available resources.

This work deals with the analysis of a specific case, namely, an industrial engineering program, and allows for the possibility of implementing this study for other academic programs in order to arrive at a general model for the university. This topic is currently being reviewed in a graduate thesis at the School of Industrial Engineering and Statistics at Universidad del Valle.

This methodology is a good approximation to the problem of the installed capacity of the university. Progress can be achieved with research by identifying relationships with other resources such as, e.g., teacher-hours, laboratory facilities, libraries, cafeteria, sports center, medical service, and technological equipment, among others.

When available resources are enough to meet the needs of students who consume capacity of the academic program j, it is necessary to analyze the trend to make decisions regarding the future evolution of available capacity. In the opposite case, in which the university’s resources are underused, it is advisable to check the causes in order to take corrective actions or to allow for better load balancing of other academic programs.

FOOTNOTES

1. The authors are especially grateful to the "Virginia Gutiérrez de Pineda" program at COLCIENCIAS for providing most of the funds required for this research study and Universidad del Valle which cofounded this study, particularly the School of Industrial Engineering and Statistics for supplying the main researcher and the necessary information for the study.

2. National Ministry of Education.

BIBLIOGRAPHIC REFERENCES

1. Antunes, A. and Peeters, D. (2000). A dynamic optimization model for school network planning. Socio economic planning sciences, 34(2), 101-120.

2. Beyrouthy, C., Burke, E., Landa-Silva, D., McCollum, B., McMullan, P., and Parkes, A. (2006). The teaching space allocation problem with splitting. The 6th international conference for the practice and theory of automated timetabling. Republica Checa: Brono.

3. Burgess, T. (1996). Planning the academic’s workload: different approaches to allocating work to university academics. Higher education, 32(1), 63-75.

4. Cox, J., Blackstone, J., and Spencer, M. (1995). APICS Dictionary (8th ed.). Falls Church, VA: American production and inventory control society.

5. Domínguez, M., Álvarez, A., Domínguez, M., and Santiago, G. (1995). Dirección de Operaciones. Aspectos estratégicos en la producción y los servicios (1st ed.). España: McGraw-Hill.

6. Dridriksson, A. (2008, June). Tendencias de la Educación Superior en América Latina y el Caribe. Documento no publicado presentado en conferencia Regional de Educación Superior, Cartagena de Indias, Colombia.

7. Gil, C. and Rivadeneira, J. (2008). Modelo metodológico para determinar la capacidad instalada en un programa académico de la Facultad de Ingeniería de la Universidad del Valle. Cali, Colombia: Universidad del Valle.

8. Greenleaf, N. and Harrison, T. (1987). A mathematical programming approach to elementary school facility decisions. Socio-economic planning sciences, 21(6), 395-401.

9. Henig, M. and Gershak, Y. (1986). Dynamic capacity planning of public schools in changing urban communities. Socio-economic planning sciences, 20(5), 319-324.

10. Instituto Latinoamericano de Liderazgo. (2010). Evolución de la Educación Superior en Colombia en la última década. Recuperado el 21 de septiembre de 2010, de http://www.universidad.edu.co/index.php?option=com_content&task=view&id=35&Itemid=96

11. Johnson, D. (2001). Lessons learned from industry: applying capacity planning in an institution for higher education. Managerial finance, 27(5), 17-32.

12. Kalenatic, D. (2001). Modelo integral y dinámico para el análisis, planeación, programación y control de las capacidades productivas en empresas manufactureras (1st ed.). Bogotá: Centro de Investigaciones y Desarrollo Científico, UDFJC.

13. Ministerio de Educación Nacional (MEN), República de Colombia. (2004). La revolución educativa 2002-2006. Recuperado en junio de 2004, de http://www.mineducacion.gov.co/1621/articles-104306_archivo_pdf.pdf

14. Ministerio de Educación Nacional (MEN), República de Colombia. (2005). Acta de Evaluación Invitación Pública MEN-28-05. Recuperado en junio de 2007, de http://menweb.mineducacion.gov.co/contratos/pdfs/evaluacion_men28.doc

15. Ministerio de Educación Nacional (MEN), República de Colombia. (2009). Sistema Nacional de Información de Educación Superior (SNIES). Recuperado en febrero de 2009, de http://200.41.9.227:7777/men/

16. Orejuela, J.P., Ocampo, J.J., and Mican, C.A. (2010). Propuesta metodológica para la programación de la producción en las pymes del sector artes gráficas, área publi-comercial. Estudios Gerenciales, 26(114), 97- 118. Recuperado de http://www.icesi.edu.co/biblioteca_digital/bitstream/10906/2238/6/5juan_orejuela_propuesta_metodologica.pdf

17. Pizzolato, N. (1994). A heuristic for large size p-median location problems with application to school location. Annals of operations research, 50(1), 473-485.

18. Tewari, V. and Jena, S. (1987). High school location decision making in rural India and location-allocation models. En A. Ghosh y G. Rushton (Eds.), Spatial analysis and location-allocation models (pp. 137-162). New York, NY: Van Nostrand Reinhold.

19. UNESCO. (2005). Hacia las sociedades del conocimiento. Paris: Autor.

20. Universidad del Valle, Oficina de Planeación y Desarrollo Institucional. (2009). Cifras. Recuperado en febrero de 2009, de http://planeacion.univalle.edu.co/a_gestioninformacion/univalle_cifras/

21. Viegas, J. (1987). Short and midterm planning of an elementary school network in a suburb of Lisbon. Sistemiurbani, 1, 57-77.

22. Yu-Lee, R. (2002). Essentials of capacity management. New York, NY: John Wiley & Sons.